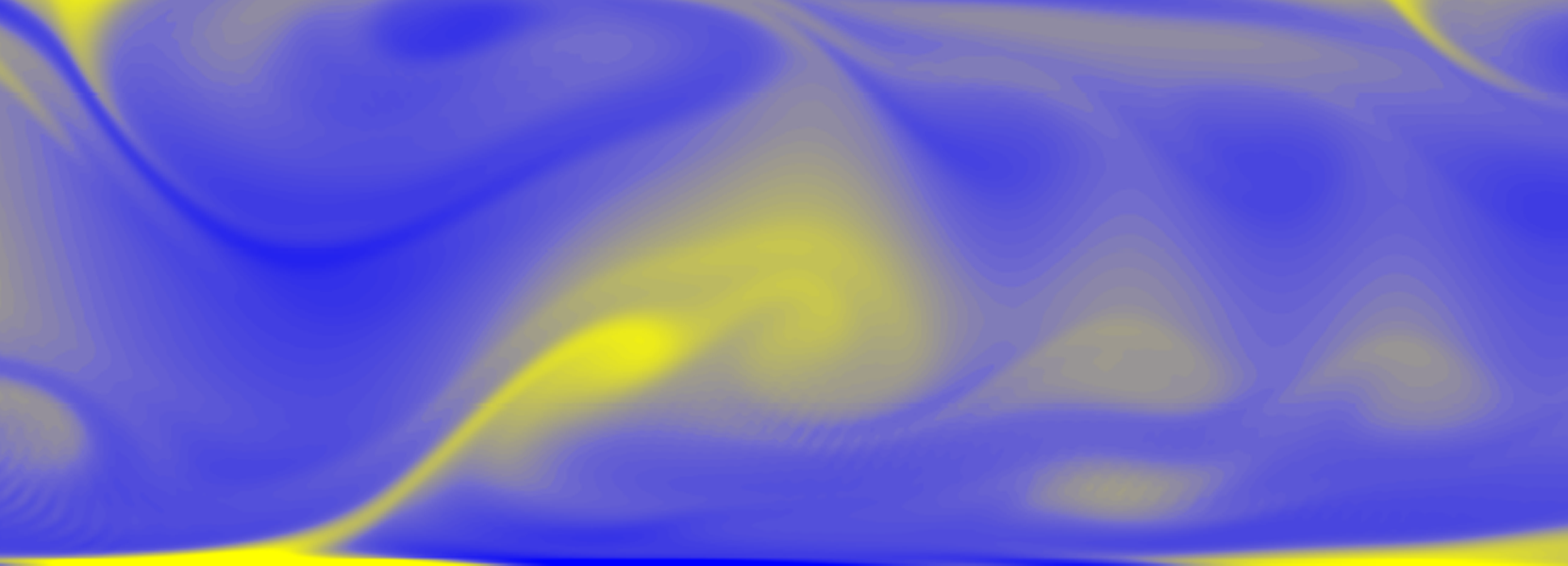
Edge states alternation and period doubling cascades in subcritical Taylor-Couette flow
Please login to view abstract download link
Coexistence of both laminar and non-trivial stable attractors is one of the main features in subcritical transition to turbulence in shear flows. By studying the Taylor-Couette flow, the fluid flow between independently rotating coaxial cylinders, we study the process by which the boundary between the two attractors acquires sensitivity to the initial conditions. In addition, when studying the non-trivial stable chaotic attractor, we analyse a period-doubling cascade using a one-dimensional discrete map reduction that confirms Feigenbaum's universal theory accurately.